How Does Limit Comparison Test Work

The limit comparison test shows that the original series is convergent. Because 1 is a finite positive number we are in case i of the limit comparison test.

The Limit Comparison Test Youtube

The limit comparison test does not apply because the limit in question does not exist.

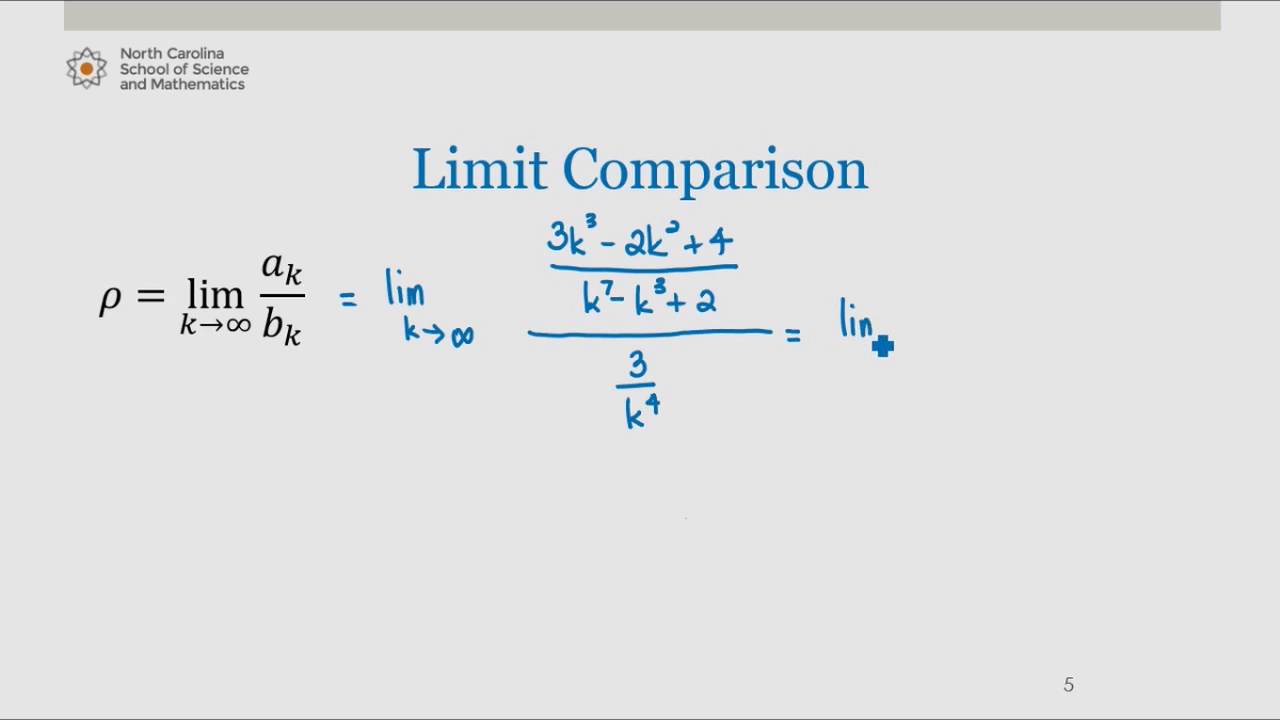
. Because our limit converged to a nite non-zero constant we may. Lim x1 fx gx lim x1 xx2 p x 1 1x lim x1 x2 x2 p x 1 1. Where a n 0 and b n 0.

Indeed lim n1 a n b n lim n1 np 21sinn 7 51 pn2 n7 lim n1 1 1 n2 sinn q 1 1 n5 n7 100 p 100 1. If the test does not apply say so. Thats why there are so many of them.

The idea behind the limit comparison test is that if you take a known convergent series and multiply each of its terms by some number then that new series also converges. Thus the LCT tells us that R 1 2 fxdxmust also diverge. Using the Ratio Test The real utility of this test is that one need not know about another series to deter-mine whether the series under consideration converges.

The limit comparison test does not apply because the limit in question does not exist. To apply the limit comparison test we must compute a limit. The limit comparison test by contrast says that if the limit you calculated is some positive real number then both integrals converge or both diverge.

The limit comparison test shows that the original series is convergent. The comparison test can be. On top of that we will need to choose the new series in such a way as to give us an easy limit to compute for c.

An converges by the limit comparison test. If your limit is non-zero and finite the sequences behave similarly so their series will behave similarly as well. Since the limit is 1 which is not 0 or 1 we can apply the LCT.

If 0 a n b n for all n N for some N then 1. If we relax the second condition to allow lim_n to infty a_nb_n 0 then we also have. If X n1 a n diverges then so does X n1 b n.

For each of the following series use the limit comparison test to determine whether the series converges or diverges. 7 What values of a makes the series converge. Multiply by the reciprocal of the denominator.

Note that both functions are positive on 11. If c is positive and is finite then either both series converge or both series diverge. Divide every term of the equation by 3 n.

The limit comparison test shows that the original series is divergent. What is limit testing. The integral R 1 2 1 x dxdiverges by the p-test with p 1.

Since the limit you calculated is 1 which is positive the hypothesis of the test is satisfied and the correct conclusion is that your two integrals either both converge or both diverge. The comparison test can be. The Limit Comparison Test.

And it doesnt matter whether the multiplier is say 100 or 10000 or 110000 because any number big or small times the finite sum of the original series is still a finite number. Sometimes the conclusion you draw is inconclusive. If X n1 b n converges then so does X n1 a n.

If lim n a n b n L where L is finite and L 0 then the two series X a n and b n either both converge or both diverge. But I get a real number N when comparing the known divergent series sumlimits_n1 frac1n with sumlimits_n1 frac1nn. Then clim n goes to infinity a nb n.

To use the limit comparison test we need to find a second series that we can determine the convergence of easily and has what we assume is the same convergence as the given series. Suppose a n 0 and b n 0 for all n. Limit tests arent guaranteed to to work.

By picking a suitable B usually a p-series we can use this test to determine whether or not A converges. Displaystyle sum_n1dfrac1sqrtn1 displaystyle sum_n1dfrac2n13n. This is very different than with the comparison tests or the integral test where some sort of comparison to another series is required.

Let n 1 a n and n 1 b n be positive-termed series. The limit comparison test is the way to formalize this intuition. L lim n 12 n n 1 n lim n 12 n 1 n 12 1 12 1 L lim n 12 n n 1 n lim n 12 n 1 n 12 1 12 1.

Lim n a n b n c where c is finite and c 0. P 1 n1 np 21sin n7n51 and P 1 n1 1 n 3 2 either both converge or both diverge. Since the numerator and denominator have the same degree the limit is simply the ratio of the x2 coe cients.

Direct Comparison Test for Series. The limit comparison test shows that the original series is divergent. After using the fact from above we can see that the Root Test tells us that this series is divergent.

R The limit comparison test is used when we want to determine whether an improper integral 1 a fxdxconverges but the function fx is too complicated for us to either compute an. Ie if the ratio of the terms tends to a finite number as n goes to infinity then both series converge or both series diverge. The limit comparison test requires that both suma_n and sumb_n have strictly positive terms and also that lim_n to infty a_nb_n 0.

Limit test is defined as quantitative or semi quantitative test designed to. 4 What values of p make the following series converge. This is a mystery mouseketool that will help us later Comparison Test.

In the limit comparison test you compare two series Σ a subscript n and Σ b subscript n with a n greater than or equal to 0 and with b n greater than 0. Using the Limit Comparison Test. Heres the limit for this series.

How does the limit comparison test work. The limit comparison test does not prove this fact.

Limit Comparison Test Youtube

The Limit Comparison Test Youtube

Limit Comparison Test Youtube